

In the modern era of minimally invasive cataract surgery, often using premium IOLs and femtosecond lasers, achieving a specific postoperative refraction is as important as addressing the cataract itself. The generally accepted goal for a premium IOL is to be within 0.50 D of emmetropia, but large studies have shown that this goal is only achievable about 70% of the time with any one unoptimized formula.1,2 This means that 30% of patients will need spectacles or more surgery to address their residual refractive error.
Cataract surgeons need to maximize accuracy and minimize postoperative refractive misses in order to improve patients’ satisfaction and decrease money spent on additional surgical procedures to refine the postoperative refraction. Certainly, ophthalmologists can optimize a specific formula for their own hands, their techniques, and their biometric equipment, but we would argue that these steps are not sufficient. We recommend changing the process of IOL calculations by selecting the best formula for each specific eye.
CHOOSING THE BEST PARTS
The current theoretical formulae such as Holladay I, SRK/T, and Hoffer Q have been the mainstay in IOL calculations for the past 20 years. These sophisticated formulas are far better than the previous regression formulae, but each has limitations under specific circumstances.
That is why John Ladas, MD, PhD, suggests optimizing multiple formulae and choosing the best parts of each of them. He has developed a two-phased approach: the Ladas super surface and Ladas super formula. The idea is to weed out the formulae’s limitations, implement adjustments, and further optimize the values over time using both individual and crowdsourced data.
APPLYING THE LADAS APPROACH
We have described the Ladas approach to IOL calculations.3 We looked at five formulas (Hoffer Q, Holladay I, Holladay I with Koch adjustment, Haigis, and SRK/T) as mathematical equations with the potential to be graphed on the x-, y-, and z-axes, and we rendered them in three dimensions one by one. By doing so, we could visualize these formulas in a manner that we had not seen before, and we could disassemble and reassemble them just how we wanted. We could then pick out the best portions of each one and incorporate them into a newly formed, singular Ladas super surface, from which we derived the Ladas super formula.
The next evolution is to include more formulae and to hone the accuracy further. All prior formulae and any future formulae can be incorporated into the Ladas super formula concept if we have access to the exact math. Although the third-generation formulae such as the Holladay 1 have been published, the newer Holladay 2 formula is proprietary and has not been publicly revealed.
OPTIMIZING THE SUPER FORMULA
The various optimizations that have already taken place are specific to or dependent on the formula used, so the optimization is only useful to eyes that are suitable for a given formula. It would be ideal to have a formula-independent optimization. We can achieve this by optimizing every millimeter of the Ladas super surface, and thus the Ladas super formula, based on empirical outcome data and by embedding other parameters (such as anterior chamber depth [ACD]) in a specific manner.
Now that we have a shape of the best of what we have so far, we can essentially sculpt and mold this shape into one that yields the most valid values. We do this primarily by comparing predicted and actual results of IOL power. This approach can evolve over time and become a system that never ceases to improve. Crowdsourcing group data means that we could use thousands, or even millions, of data points to achieve unprecedented accuracy.
EXAMPLES
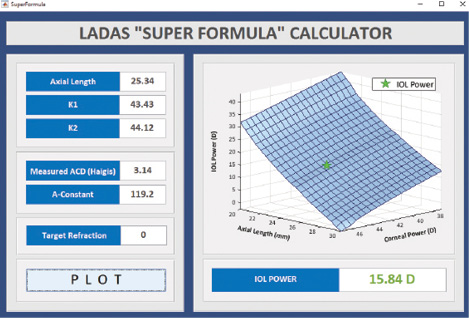
Figure 1. A standard eye: axial length 25.34 mm; K1 43.43 D, K2 44.12 D; ACD 3.14 mm; and target refraction plano. Abbreviations: K, keratometry; ACD, anterior chamber depth.
Here are a few examples of how the super formula offers calculation options for a diversity of eyes. In a standard eye, the super formula interface localizes to the correct region and provides the most accurate IOL power value. The interface takes into account the axial length, keratometry, A-constant, target refraction, and measured ACD, if needed (Figure 1).
In eyes with a short axial length, the slightest change in effective lens position can dramatically change calculation results. We have found that the super formula easily locates the correct region on the super surface and provides the most accurate calculation (Figure 2).
IOL power calculations are often inaccurate in eyes with an axial length greater than 26 mm unless the ophthalmologist applies the Koch adjustment. The super formula automatically takes this adjustment into account (Figure 3).
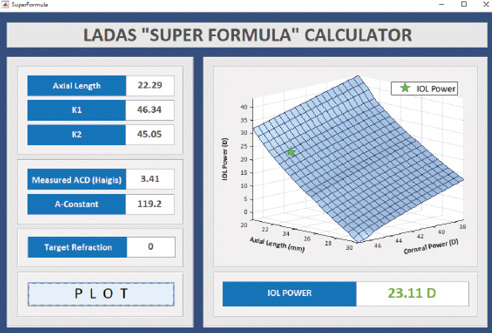
Figure 2. A small eye: axial length 22.29 mm; K1 46.34 D, K2 45.05 D; ACD 3.41 mm; and target refraction plano.
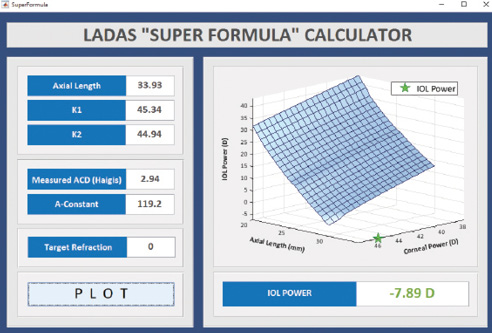
Figure 3. A long eye: axial length 33.93 mm; K1 45.34 D, K2 44.94 D; ACD 2.94; and target refraction plano.
CONCLUSION
We believe that the Ladas super formula can streamline the selection of the most appropriate IOL formula for a given eye and improve outcomes. By viewing the current IOL formulae as 3-D entities, we can choose the best portions of each public formula to calculate the most appropriate IOL power value for eyes with any axial length, keratometry reading, and ACD. As surgeons continue to optimize their approach, the most important goal is to achieve better outcomes for patients and inch closer to the dream of spectacle independence. They may learn more or try the formula as a beta tester at www.IOLcalc.com.
1. Aristodemou P, Knox Cartwright NE, Sparrow JM, Johnston RL. Formula choice: Hoffer Q, Holladay 1, or SRK/T and refractive outcomes in 8108 eyes after cataract surgery with biometry by partial coherence interferometry. J Cataract Refract Surg. 2011;37(1):63-71.
2. Gale RP, Saldana M, Johnston RL, et al. Benchmark standards for refractive outcomes after NHS cataract surgery. Eye (Lond). 2009;23(1):149-52.
3. Ladas JG, Siddiqui AA, Devgan U, Jun AS. A 3-D “super surface” combining modern intraocular lens formulas to generate a “super formula” and maximize accuracy. JAMA Ophthalmol. 2015;133(12):1431-1426.
Uday Devgan, MD
• private practice, Devgan Eye Surgery, Los Angeles
• clinical professor of ophthalmology, Jules Stein Eye Institute, UCLA
• chief of ophthalmology, Olive View UCLA Medical Center
• (800) 377-1969; devgan@gmail.com; Twitter @devgan
• financial disclosure: principal in Advanced Euclidean Solutions, which owns the Ladas super formula, Ladas super surface, and associated material
Aazim A. Siddiqui, MD
• investigator, Advanced Euclidean Solutions, Silver Spring, Maryland
• aazim.siddiqui@gmail.com
• financial disclosure: principal in Advanced Euclidean Solutions, which owns the Ladas super formula, Ladas super surface, and associated material






