Over the past decade, great strides have been made to improve the efficiency, safety, and stability of outcomes after cataract surgery. With this progress, refractive cataract surgeons can reduce astigmatism and provide spectacle independence better than ever before. To provide these refractive outcomes, surgeons rely on accurate biometric measurements and modern IOL selection formulas. This article shares our strategy for selecting the lens’ power.
AT A GLANCE
• Take multiple measurements of all of the data used for the formulas for IOL power calculation.
• Apply a data screening verification method to eliminate outliers and refine measurements.
• Apply the appropriate formulas according to the axial length of the eye measured.
PREOPERATIVE DATA POINTS
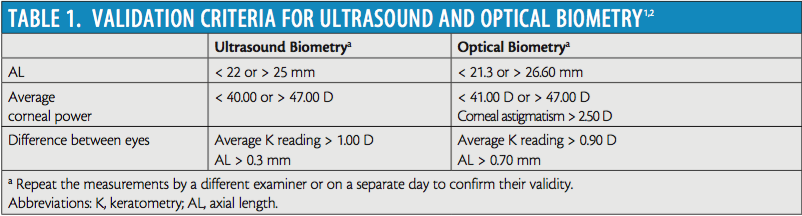
The fundamental input values for IOL formulas are ocular biometry measurements. The biometric measurements required for third-generation formulas such as Holladay 1, SRK/T, and Hoffer Q are axial length (AL) and average keratometry reading. Newer-generation formulas require additional historical information and other measurements such as age, preoperative refraction, lens thickness, and anterior chamber depth. To ensure that accurate measurements have been obtained before surgery, we repeat multiple measurements of the eye to gather a valid sample of data. This step is the core of our strategy. Once we have captured multiple measurements, we apply screening validation criteria that have been developed for both ultrasound and optical biometry to detect potential errors in gross measurement. Table 1 summarizes these criteria.1,2 Measurements that fall outside these ranges need to be repeated by a different examiner or on a separate day to confirm validity.
It is also important to integrate data from the clinical examination and other diagnostics to ensure the validity of the biometry measurements. For example, keratometry is dependent on reflections from the tear film to produce accurate measurements. Disruptions to the tear film affect keratometry, so we keep in mind the effect that dry eye disease (particularly fast tear breakup time) or anterior basement membrane dystrophy may have on the corneal power measurement.
Topographies are frequently obtained in the context of toric IOL planning. We recommend reviewing the topography to ensure that there is no irregular astigmatism from sources such as anterior basement membrane dystrophy or ectasia. Doing so can help increase the surgeon’s confidence in using the keratometry measurements. When the automated keratometry value has a large standard deviation, it is important to consider these clinical entities.
ACCURACY
Once we are comfortable with the measurements we have captured preoperatively, the accuracy of the numerous formulas available becomes a factor. Most current third-generation formulas (SRK/T, Holladay 1, Hoffer Q) accurately determine the effective lens positions (ELPs) required for a strong refractive outcome using AL and keratometry in normal (72% of eyes are 22-24.5 mm in length) and mildly myopic eyes (15% of all eyes are between 24.5 and 26 mm in length).3 When we try to apply the same ELP predictions to the 13% of eyes that fall outside this range, postoperative refraction predictions become less accurate. This limitation is evident in some large-scale studies that conclude third-generation formulas are able to achieve a mean ±0.50 D prediction accuracy between 70% and 80%.4,5 Modifications to the formulas or adopting newer-generation formulas that use additional measurement values will improve predictions.
AL Adjustment Method for Myopes
In cases of moderate to extreme axial myopia (> 26 mm; 7% of eyes3), we have found that the accuracy of outcomes can be improved by modifying the AL value entered in third-generation formulas. Wang and colleagues stated that highly myopic eyes have much more vitreous liquefaction, which changes the refractive index compared to normal eyes.6 Unfortunately, current optical biometers use an average refractive index to measure the entire eye. Eyes longer than 26 mm may deviate from this “normal” average refractive index, and as a result, AL measurements in these long eyes can be inaccurate.6
Wang et al developed a nomogram based on their data and those from an independent second center to adjust the AL measurements to account for the hyperopic drift in these extremely myopic eyes:
•Holladay 1 two-center optimized AL = 0.8814 × IOLMaster (Carl Zeiss Meditec) AL + 2.8701
•Haigis two-center optimized AL = 0.9621 × IOLMaster AL + 0.6763
•SRK/T two-center optimized AL = 0.8981 × IOLMaster AL + 2.5637
•Hoffer Q two-center optimized AL = 0.8776 × IOLMaster AL + 2.9269
In a retrospective study by Abulafia et al, this method of AL adjustment was compared to unadjusted versions of the same formulas as well as newer formulas not requiring AL adjustment such as the Holladay 2, Barrett Universal II, and Olsen.7 For the most challenging group of eyes with an AL greater than 26 mm and where an IOL power of less the 6.00 D was used, the Barrett Universal II, AL-adjusted Haigis, and AL-adjusted Holladay 1 formulas all met benchmark criteria. In this same group, the mean absolute error was the lowest with the Barrett Universal II and all three AL-adjusted formulas (not statistically significantly different). When the AL was greater than 26 mm and the IOL power was greater than 6.00 D, the AL-adjusted formulas overcompensated, which resulted in a slight overcorrection. In this less challenging group, the SRK/T, Hoffer Q, Haigis, Barrett Universal II, Holladay 2, and Olsen all met benchmark criteria.
Hyperopic Eyes
In hyperopic eyes (AL < 22 mm, 8%),3 accurate measurements and ELP predictions become even more important. The IOL power recommendations in these eyes tend to be much greater than in myopic eyes, so inaccuracies in ELP or IOL power recommendations can lead to greater prediction errors than in myopic eyes. In a study by MacLaren et al, eyes measured with optical biometry and requiring IOL powers from 30.00 to 35.00 D had the smallest prediction errors when the Haigis (optimized on the IOLMaster for one constant) or the Hoffer Q formula was used.8
CONCLUSION
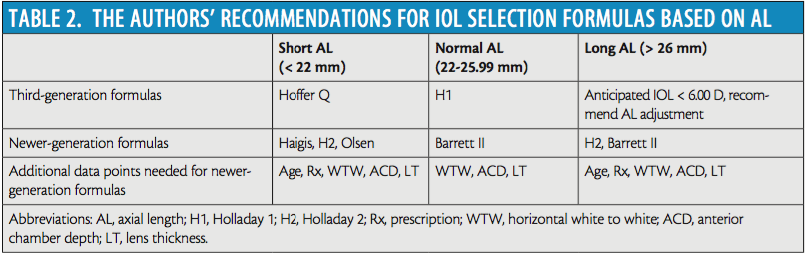
No single formula has the best prediction error across the varying ranges of AL of eyes on which we perform cataract surgery. Table 2, which is based on published data and our personal outcomes, outlines the formulas we use. The table is organized into third-generation formulas and the newer formulas that require more data. We encourage surgeons to review their own outcomes, optimize/personalize constants and factors, and see which formulas are the most accurate for their practices.
In their efforts to improve predictive accuracy, surgeons should continue to evaluate newer formulas and technologies as they become available. As newer formulas have been introduced, predictive accuracy has steadily increased (able to achieve a mean ±0.50 D accuracy: Holladay 1, 88.3%; Haigis, 88.7%; Holladay II, 89.2%; Olsen, 90.9%; Barrett II, 91.5% [W.E. Hill, personal communication, February 2016]). Early trials of a new formula, the radial basis function formula, have achieved a mean ±0.50 D accuracy of 95% for 3,212 cases across 13 surgeons in eight countries (W.E. Hill, personal communication, February 2016).
Other strategies include using intraoperative aberrometry to help improve on the preoperative planning formula outcomes. In addition, surgeons may look at newer IOLs to correct for errors in prediction. For example, the Light Adjustable Lens (Calhoun Vision; not FDA approved) may change the postoperative refraction of the patient from -2.00 to +2.30 D and correct up to 2.60 D of astigmatism after implanted in the eye.9
With multiple strategies to help ophthalmologists achieve their goals, the future is bright for improving outcomes after cataract surgery.
1. Holladay JT, Prager TC, Chandler TY, et al. A three-part system for refining intraocular lens power calculations. J Cataract Refract Surg. 1988;14:17-24.
2. Knox Cartwright NE, Johnston RL, Jaycock PD, et al. The Cataract National Dataset electronic multicentre audit of 55,567 operations: when should IOLMaster biometric measurements be rechecked? Eye. 2010;24(5):894-900.
3. Fotedar R, Wang JJ, Burlutsky G, et al. Distribution of axial length and ocular biometry measured using partial coherence laser interferometry (IOLMaster) in an older white population. Ophthalmology. 2010;117(3):417-423.
4. Wang L, Shirayama M, Ma XJ, et al. Optimizing intraocular lens power calculations in eyes with axial lengths above 25.0 mm. J Cataract Refract Surgery. 2011;37(11):2018-2027.
5. Abulafia A, Barrett GD, Rotenberg M, et al. Intraocular lens power calculation for eyes with an axial length greater than 26.0 mm: comparison of formulas and methods. J Cataract Refract Surgery. 2015;41(3):548-556.
6. MacLaren RE, Natkunarajah M, Riaz Y, et al. Biometry and formula accuracy with intraocular lenses used for cataract surgery in extreme hyperopia. Am J Ophthalmol. 2007;143(6):920-931.
7. Hahn U, Krummenauer F, Kölbl B, et al. Determination of valid benchmarks for outcome indicators in cataract surgery: a multicenter, prospective cohort trial. Ophthalmology. 2011;118(11):2105-2112.
8. Behndig A, Montan P, Stenevi U, et al. Aiming for emmetropia after cataract surgery: Swedish National Cataract Register study. J Cataract Refract Surg. 2012;38(7):1181-1186.
9. Villegas EA, Alcon E, Rubio E, et al. Refractive accuracy with light-adjustable intraocular lenses. J Cataract Refract Surg. 2014;40(7):1075-1084.e2.
Anthony Kuo, MD
• assistant professor of ophthalmology, Duke University Eye Center, Durham, North Carolina
• anthony.kuo@dm.duke.edu
• financial disclosure: consultant to Alcon and ClarVista Medical
Robin Raul Vann, MD
• assistant professor of ophthalmology, Duke University Eye Center, Durham, North Carolina
• robin.vann@duke.edu
• financial disclosure: consultant and research consultant to Alcon; consultant to ClarVista Medical